Yesterday I got started on the first component: getting audio into the microcontroller. I want to start with a standard dynamic mic, so I can keep using the same mic for talkbox and whistle synth, so it should take standard balanced audio on XLR as input. In a full version this would need an XLR port, but for now I can pull back the housing on an XLR cable:
Or, if you're prefer a different model:
Since last time, I:
-
Fixed my interference issues by:
- Grounding the piezo input instead of putting it at +1.65v.
- Shielding my longer wires.
- Soldering my ground and power pins that I'd missed initially (!!)
Got the software working reasonably reliably.
-
Designed and 3D printed a case, with lots of help from my MAS.837 TA, Lancelot.
-
Dumped epoxy all over the back of the metal plate to make it less likely the little piezo wires will break off.
Revived the Mac version of my MIDI mapper and made a cut down version that is designed to drive a wind instrument synth (code).
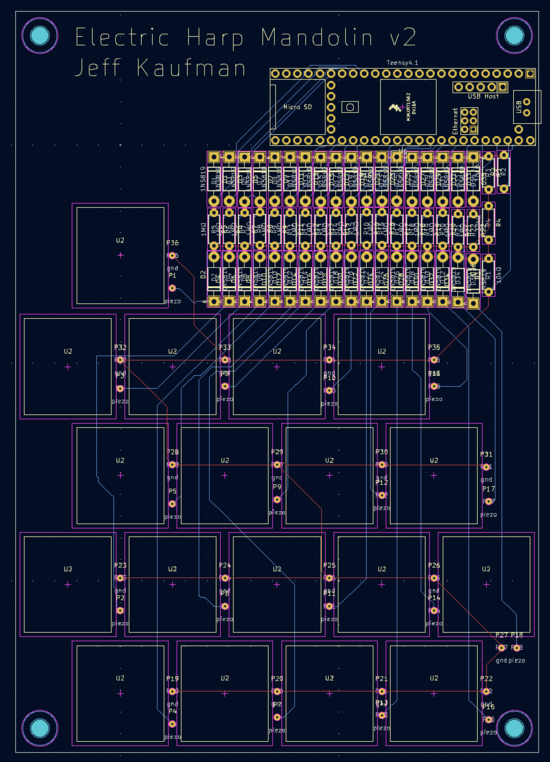
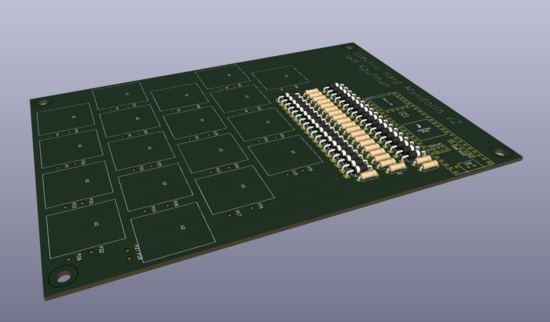
While this works, it's a little bulky for attaching to the mandolin. I also don't like having a bunch of wires running from the teeth to the computer and that I only have thirteen of the eighteen inputs hooked up. I've also been enjoying playing it with my fingers, in more of a piano orientation. I decided to make a new version that's all a single board:
The squares show where the "teeth" will go.
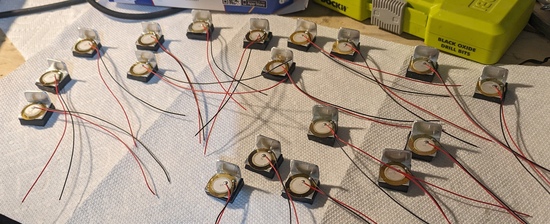
I've prepared a new batch of eighteen pluck sensors:
Sanding them smooth was a good fit for watching the kids at the park.
I'm a bit nervous with this design that something will break and it will be hard to repair because it's all on one board, but it's got to be better than the giant blob of epoxy in the v1 design.
If this works well I might make a case for it where only the teeth poke out?
This post uses the same approach as last year, which is almost the same as before then. Numbers are monthly, based on 2023 spending:
| Work | Nucleic Acid Observatory | |
| Work | Speaking | |
| Band | Free Raisins | |
| Band | Kingfisher | |
| Code | Whistle Synth | |
| Code | Apartment Price Map | |
| Board | BIDA Contra | |
| Board | Giving What We Can | |
| Child | Lily | |
| Child | Anna | |
| Child | Nora |
Main Topics (all)